College Algebra
Answer/Discussion to Practice Problems
Tutorial 41: Practice Test on Tutorials 34 - 40
Problems 1a - 1b: Find the coordinates of the
vertex of the
given quadratic function. Without graphing, determine if the
vertex
is the maximum or minimum point of the quadratic function. |
1a. 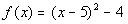
 Answer: Answer:
VERTEX
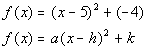
Since (h, k) is the vertex in standard form
then the vertex is
(5, -4).
MAX OR MIN?
Since a = 1, and 1 is greater than 0,
this
parabola would open up.
So our vertex (5, -4) is the minimum point. |
|
1b. 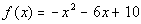
 Answer: Answer:
VERTEX
Using the vertex formula
we get:
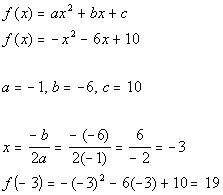
The vertex would be (-3, 19)
MAX OR MIN?
Since a = -1, and -1 is less than 0,
this
parabola would open down.
So our vertex (-3, 19) is a maximum point. |
|
Problems 2a - 2b: Use the vertex and the
intercepts to sketch
the graph of the given quadratic function. Find the equation for
this function’s axis of symmetry. |
2a. 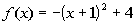
 Answer: Answer:
CURVE
Since a = -1 and -1
< 0, then it
looks like it is going to curve down.
VERTEX
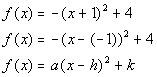
Vertex is (-1, 4)
INTERCEPTS
y-intercept:
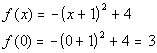
y-intercept: (0,
3)
x-intercept:
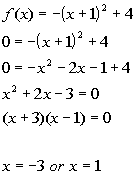
x-intercepts:
(-3, 0) and (1, 0)
GRAPH
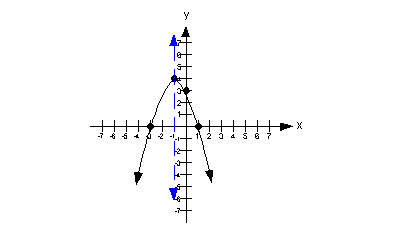
As shown on the graph, the axis of symmetry is x = -1. |
|
2b. 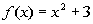
 Answer: Answer:
CURVE
Since a = 1 and 1
> 0, then it looks
like it is going to curve up.
VERTEX

Vertex is (0, 3)
INTERCEPTS
y-intercept:
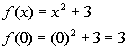
y-intercept: (0,
3)
x-intercept:
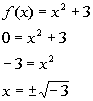
x-intercepts:
Note how we got a negative number underneath the square root.
That means there is no real number solution. That also means
that
there are NO x- intercepts.
GRAPH
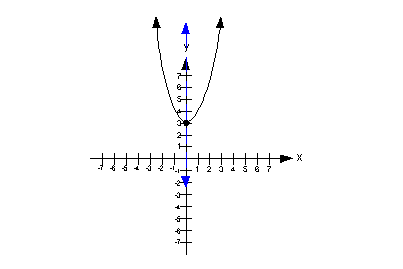
As shown on the graph, the axis of symmetry is x = 0. |
|
Problem 3a: Given the polynomial function a)
use the Leading
Coefficient Test to determine the graph’s end behavior, b) find the
x-intercepts
(or zeros) and state whether the graph crosses the x-axis or touches
the
x-axis and turns around at each x-intercept, c) find the y-intercept,
d)
determine the symmetry of the graph, e) indicate the maximum possible
turning
points, and f) graph. |
3a. 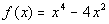
 Answer: Answer:
a) LEADING COEFFICIENT
TEST
Since the degree of the polynomial, 4, is
even and the leading
coefficient, 1, is positive, then the graph of the given polynomial
rises
to the left and rises to the right.
b) X-INTERCEPT
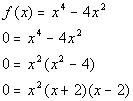
First factor:
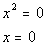
Since the exponent on this factor is 2, then
multiplicity for the zero x = 0 is 2.
Since the multiplicity is 2, which is even,
then the graph touches
the x-axis and turns around at the zero x =
0.
Second factor:
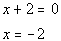
Since the exponent on this factor is 1, then
multiplicity for the zero
x = -2 is 1.
Since the multiplicity is 1, which is
odd, then the graph crosses
the x-axis at x = -2.
Third factor:
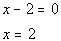
Since the exponent on this factor is 1, then
multiplicity for the zero x = 2 is 1.
Since the multiplicity is 1, which is
odd, then the graph crosses
the x-axis at x = 2.
c) Y-INTERCEPT
Letting x = 0 we
get:
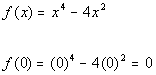
The y-intercept
is (0, 0).
d) SYMMETRY
Y-axis symmetry:
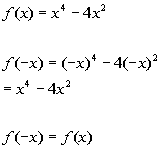
It is symmetric about the y-axis.
Origin symmetry:
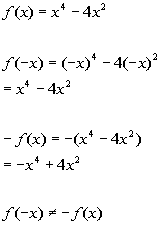
Is not symmetric about the origin.
e) TURNING POINTS
Since the degree of the function is 4, then there
is at most 4 -
1 = 3 turning points.
EXTRA POINTS
To get a more accurate curve, lets find some
points that are in
between the points we found above:
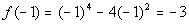
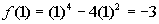
So (-1, -3) and (1, -3) are two more points on
the graph.
f) GRAPH
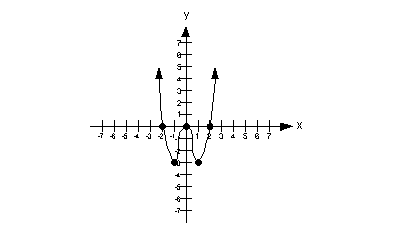
|
|
Problem 4a: Divide using long division. |
4a. 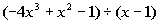
|
Problem 5a: Divide using synthetic division. |
5a. 
|
Problem 6a: Given the function f(x),
use the Remainder Theorem to find f(-2). |
Problem 7a: Solve the given equation given
that 2/3 is a zero
(or root) of 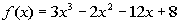 . . |
7a. 
 Answer: Answer:
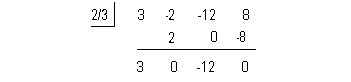
Rewriting f(x)
as (x - 2/3)(quotient) we get:
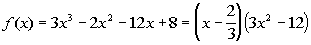
Setting f(x)
= 0 and solving we get:
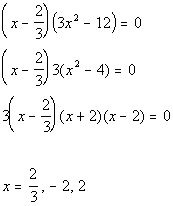 |
|
Problems 8a - 8b: List all of the possible
zeros or roots,
use Descartes’ Rule of Signs to possible narrow it down, use synthetic
division to test the possible zeros or roots and find an actual zero or
root, and use the actual zero to find all zeros or the actual root to
find
all solutions to the given polynomial function or equation to solve. |
8a. 
 Answer: Answer:
POSSIBLE ZEROS
The factors of the constant term -6 are . .
The factors of the leading coefficient 1 are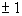 . .
Writing the possible factors as p/q we get:

DESCARTES' RULE OF SIGNS
Possible number of positive real zeros:
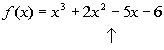
The up arrows are showing where there are sign changes between
successive
terms, going left to right.
There is 1 sign change between successive terms,
which means there
is exactly one positive real zero.
Possible number of negative real zeros:
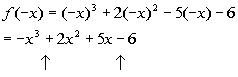
There are 2 sign changes between successive terms,
which means that
is the highest possible number of negative real zeros. To find
the
other possible number of negative real zeros from these sign changes,
you
start with the number of changes, which in this case is 2, and then go
down by even integers from that number until you get to 1 or 0.
Since we have 2 sign changes with f(x), then there
are possibility
of 2 or 2 - 2 = 0 negative real zeros.
FIND A ZERO
I’m going to choose -1 to try:
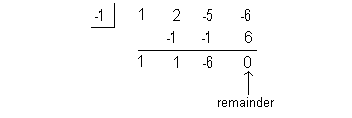
We found a number that has a remainder of 0. This
means that x = -1
is a zero or root of our polynomial function.
FIND OTHER ZEROS
Since, x = -1 is a zero, that means x + 1 is a factor of our polynomial function.
Rewriting f(x)
as (x + 1)(quotient) we get:
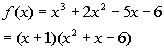
We need to finish this problem by setting this
equal to zero and solving
it:
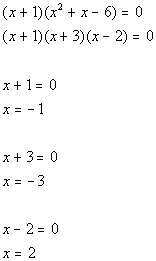
The zeros of this function are x =
-3, -1, and 2. |
|
8b. 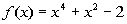
 Answer: Answer:
POSSIBLE ZEROS
The factors of the constant term -2 are . .
The factors of the leading coefficient 1 are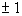 . .
Writing the possible factors as p/q we get:
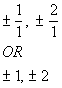
DESCARTES' RULE OF SIGNS
Possible number of positive real zeros:
The up arrows are showing where there are sign changes between
successive
terms, going left to right.
There is 1 sign change between successive terms,
which means there
is exactly one positive real zero.
Possible number of negative real zeros:
There is 1 sign change between successive terms,
which means there
is exactly one negative real zero.
FIND A ZERO
I’m going to choose 1 to try:
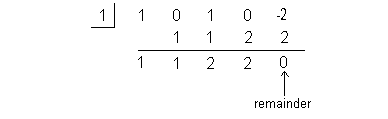
We found a number that has a remainder of 0. This
means that x = 1 is
a zero or root of our polynomial function.
FIND OTHER ZEROS
Since, x = 1 is a zero, that means x - 1 is a factor of our polynomial function.
Rewriting f(x)
as (x - 1)(quotient) we get:
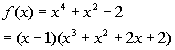
We need to finish this problem by setting this
equal to zero and solving
it:
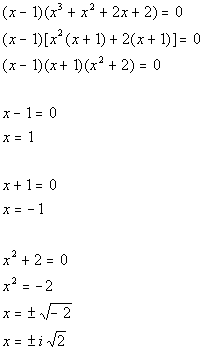
The zeros of this function are x =
-1, 1, 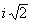 , , 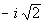 . . |
|
Problem 9a: Show that all real roots of the
given equation
lie between -2 and 2. |
9a. 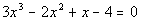
 Answer: Answer:
LOWER BOUND
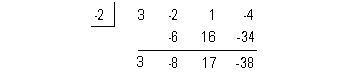
Since c = -2 < 0
AND the successive signs
in the bottom row of our synthetic division alternate, then -2 is a
lower
bound for the real roots of this equation.
UPPER BOUND
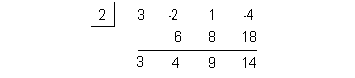
Since c = 2 > 0
AND all of the signs
in the bottom row of our synthetic division are positive, then 2 is an
upper bound for the real roots of this equation.
Since -2 is a lower bound and 2 is an upper bound
for the real roots
of the equation, then that means all real roots of the equation 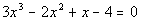 lie between -2 and 2. lie between -2 and 2. |
|
Problem 10a: Show that the given polynomial
has a real zero
between the given integers. Use the Intermediate Value theorem to
find an approximation for this zero to the nearest tenth. |
10a. 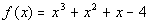 ;
between 1 and 2.
 Answer: Answer:
Finding f(1): 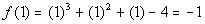
Finding f(2): 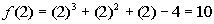
Since there is a sign change between f(1)
= -1 and f(2) = 10, then according to
the Intermediate
Value Theorem, there is at least one value between 1 and 2 that is a
zero
of this polynomial function.
Checking functional values at intervals of
one-tenth for a sign change:
Finding f(1): 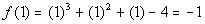
Finding f(1.1): 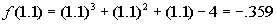
Finding f(1.2): 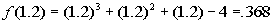
Hey we have a sign change. Now we want to
find the zero to the
nearest tenth. So is it going to be x = 1.1 or x = 1.2. We can not
necessarily
go by which functional value is closer to zero. We will need to
dig
a little bit deeper and go by intervals of one-hundredths.
Finding f(1.11): 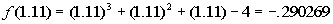
Finding f(1.12): 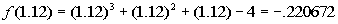
Finding f(1.13): 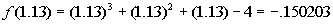
Finding f(1.14): 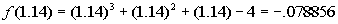
Finding f(1.15): 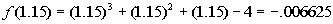
Finding f(1.16): 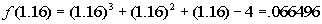
Whew!!!! At last we come to a sign change
between successive hundredths.
There is a zero between 1.15 and 1.16.
The nearest tenth would be 1.2. |
|
Problem 11a: Use the given root to find all of
the roots of
the given polynomial equation. |
11a. 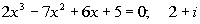
 Answer: Answer:
The complex number 2 + i is a root, that
means it’s conjugate 2 - i is also a
root.
Using synthetic division with the given zero to
find the quotient
we get:
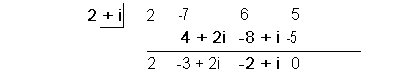
Using synthetic division with the conjugate 2 - i and the quotient found above we get:
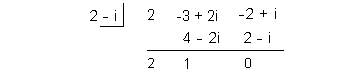
From here we can rewrite the original problem
using the roots that we
have above and the quotient that we ended up with in this last
synthetic
division.
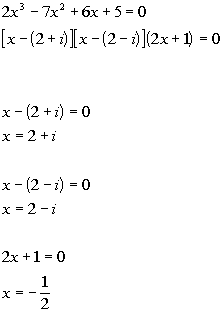
So the roots of the polynomial equation are 2 + i,
2 - i, -1/2. |
|
Problem 12a: Factor the given polynomial
function a)
as the product of factors that are irreducible over rational numbers,
b)
as the product of factors that are irreducible over real numbers, and
c)
in completely factored form involving complex nonreal numbers. |
12a. 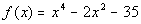
 Answer: Answer:
a) FACTORS THAT ARE
IRREDUCIBLE OVER RATIONAL
NUMBERS
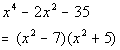
Since 7 is not a perfect square, this is as far as
we can factor it
using only rational numbers.
b) FACTORS THAT ARE
IRREDUCIBLE OVER REAL NUMBERS
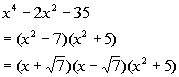
Even though 7 is not a perfect square, we can
factor the first factor
found above as a difference of squares and end up with an irrational
number,
which is still a real number.
c) COMPLETELY FACTORED
OVER COMPLEX NONREAL
NUMBERS
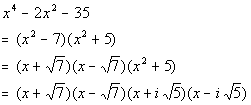 |
|
Problem 13a: Find an nth
degree
polynomial function with the given conditions. |
13a. n = 3; -3i and 2 are zeros and f(1) = -20
 Answer: Answer:
WRITE OUT ALL FACTORS OF
THE POLYNOMIAL
Since our degree is 3, that means there are three linear factors over
the complex numbers (possibly real and not necessarily distinct).
Note how we are only given two zeros. We
need to come up with
a third one.
Since -3i is a zero,
that means its conjugate,
3i, is also a zero.
Using the Linear Factorization Theorem we get: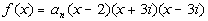
MULTIPLY FACTORS
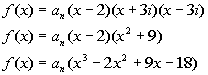
FINDING a
This problem gave another condition, f(1)
= -20.
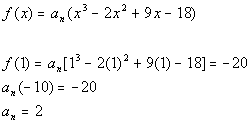
PUTTING IT ALL TOGETHER
 |
|
Problem 14a: Sketch the graph of the rational
function. |
14a. 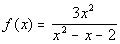
 Answer: Answer:
REDUCE

This function cannot be reduced any
further. This means that there will be no open holes on this
graph.
VERTICAL ASYMPTOTES
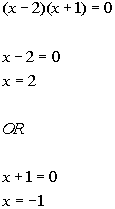
HORIZONTAL ASYMPTOTES

SYMMETRY
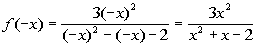
Since  , the function is neither even nor
odd. This means the graph is not symmetric about the y-axis nor the origin. , the function is neither even nor
odd. This means the graph is not symmetric about the y-axis nor the origin.
INTERCEPTS
y-intercept:
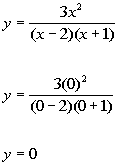
y-intercept: (0,
0)
x-intercept:
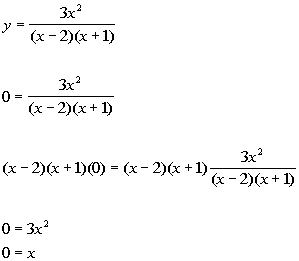
x-intercept: (0,
0)
GRAPH
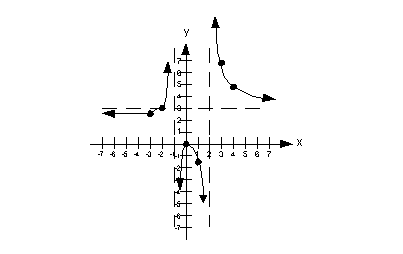
|
|

Last revised on March 20, 2011 by Kim Seward.
All contents copyright (C) 2002 - 2011, WTAMU and Kim Seward.
All rights reserved.
|
|


